Optimal Game Theory Poker
Poker has evolved immensely over the last years. One of the theories that most changed the landscape is game theory optimal poker (GTO). Nowadays, at least in high stakes poker, some GTO knowledge is necessary to become a winning player. But even for low and middle stakes poker, studying GTO basic concepts can help your game immensely!
Play Optimal Poker shatters the myth that game theory is only for elite poker players. Renowned poker pro and coach Andrew Brokos takes you step-by-step through the fundamentals, explaining core game theory principles and how to apply them in real poker. Game Theory Optimal Game Theory Optimal or else GTO is the strategy that gives out the best Expected Value (EV) against an opponent that chooses to always play with a counter-strategy from the one you used. If that sounds difficult to comprehend consider following this lesson and you will learn how to use GTO for your advantage against any player that has specifically pushes you around with.
What is game theory?
Game theory is the mathematical modeling of strategic interactions among rational decision-makers, like auction bargainers, stock exchange agents, or game players. It was popularized by “A Beautiful Mind,” a movie about the American mathematician John Nash. Nash introduced the Nash equilibrium, a solution where players, even if they know the strategy of others, can not gain by altering their tactics. Game theory has many applications in different fields, such as social science and economics.
One of the simplest examples to explain game theory is the familiar game of Rock-Paper-Scissors. In this game, a Nash equilibrium is reached when players choose at random between the three possible options, 33.3% Rock, 33.3% Paper, and 33.3% Scissors.
So, when a player applies this “solution” strategy, even if his opponent knows it, he cannot find a way of exploiting it. Other strategies that are not Nash Equilibriums have flaws that can potentially be exploited. For example, if a player has a different probability of selecting the same object twice in a row, then his strategy is not balanced. An observant opponent can adapt accordingly and take advantage of this imbalance.
What is GTO poker?
GTO poker means using game theory and Nash Equilibrium to create balanced, unexploitable strategies. Unexploitable means that, even if your opponent knew your strategy, he could not discover ways to exploit it.
For example, GTO poker can produce balanced open-raise ranges, containing value hands and light hands, so that your opponent will not be able to make easy decisions, nor will he be able to comfortably re-raise bluff you.
Is no-limit Holdem solved?
No-limit multiplayer poker is so complex that it is not entirely solved. However, some specific situations, like short-stack preflop pushing ranges or river betting ranges, can be resolved. In such cases, game theory can be useful, as it can produce Nash equilibriums that opponents cannot exploit.
Therefore, in poker, GTO can help you understand what a balanced strategy is. For example, when you open raise, your range must have the right proportion of premium and speculative hands. If your range is too broad, containing too many speculative hands, your opponents would be able to three-bet bluff you out of the pot profitably with any hand. On the other side, if your open-raise range is too narrow, your opponents would be able to put you on premium hands, and not give you enough action. GTO can provide solutions to such scenarios so that you remain unpredictable.
GTO extends to every decision you make, from preflop actions, all the way to the river. To reach a balanced strategy, you have to start thinking about your hands in terms of ranges. In any situation, you have to examine if your hand is part of a balanced mix of hands with which you would act a specific way.
When should one use GTO?
GTO is not necessarily the most profitable strategy. In particular, against weak players, using exploitative strategycan be more profitable. However, if you do not know your opponent’s playing style and leaks, you can start by playing GTO poker until you gain some intuition. GTO can also serve as a defense strategy against very skilled poker players as it is unexploitable.
In our Rock-Paper-Scissors example, if you knew that a player chose Paper more often, sticking to your balanced approach would be sub-optimal. You could select more often Scissors to increase the chances of winning him.
The same applies to poker. As weak players play far from equilibrium, by playing exploitative poker, you can better take advantage of their shortcomings. However, understanding GTO can help your game enormously! GTO can show you what a balanced strategy is. Consequently, knowing where the baseline lays can give you useful insights on when someone deviates from it and how to counter his play accordingly. For example, if you observe that a player bluffs much more frequently on the river than what GTO indicates as optimal, you can profitably call with the majority of your made hands.
GTO can also serve as a defense strategy against very skilled poker players. It can teach you how to balance your game so that opponents will not be able to exploit you.
Another way to use profitably GTO strategy is when you are playing against an unknown player. We sometimes rush into assumptions about others that often prove to be false. You cannot deduce your opponent’s playing style and betting patterns based on observing only a couple of hands. So, it is best to use a balanced strategy against an unknown player, until you gather enough insights to understand your opponent’s leaks and exploit them!
An example
Let’s take a look at a specific example and see how to use GTO.
You raise from the cut-off with K♠Q♠ and the big blind calls. Your preflop opening range in this position can be wide:
The flop comes 9♠7♦5♥. You c-bet on the flop and two-barrel on the turn, the 2♠, as you pick up a flush draw to go along with your overcards. Your opponent calls and the river is the J♠, so you make your flush!
The first thing to do is estimate the set of hands with which you would value bet in this spot. Then we can calculate how many bluffs we can incorporate to have a GTO balanced mix.
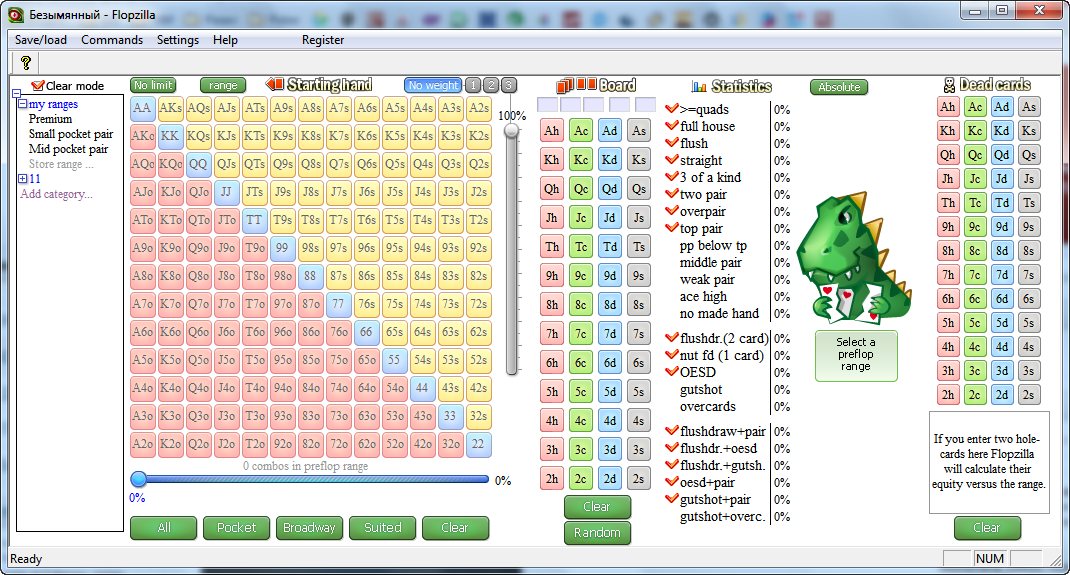
Let’s suppose that, after two barreling, you would value bet the river with an overpair or better. For simplicity, we will also consider that these hands are in accordance with your post-flop c-bets. Using Flopzilla, we can calculate that “any overpair or better” corresponds to about 16% of your opening range, and looks like this:
So, how many bluffs should you incorporate into the mix?
First, let’s consider that your value bet is pot-sized. Your opponent has to call a pot-sized bet to potentially win two times as much. So, his pot odds are 2 to 1, and he would need to win 33.3% of the time to break even. So, in this case, if your mix contains 33.3% of bluffs, it is balanced. This means that even if your opponent knew that you bluff 33.3% of the time, he would not be able to exploit it.
If you bluff more frequently than 33.3%, your opponent could exploit your strategy by profitably calling with his medium-strength hands. If you bluff less, your opponent would be able to fold his medium-strength hands, knowing you scarcely bluff!
Validation using Expected Value
To demonstrate that this strategy is balanced, we can use expected value to see if your opponent’s strategy can affect your EV, meaning if he can somehow profit against you. To simplify the analysis, since your opponent will always call with his powerful hands, and fold when he has nothing, we will examine his medium-strength hands.
If your opponent always calls with his medium-strength hands, your EV against them would be +(2*pot)*0.667 – pot*0.333 = pot
If your opponent always folds his medium-strength hands, your EV would be pot*100% = pot
If your opponent calls x% of the time, your EV would be +x*(2*pot)*0.667 – x*pot*0.333 + (1-x)*pot*100%= pot
So your opponent cannot exploit your range. Your bluffs hands should be the ones that have the smallest showdown equity. Thus, a balanced betting range would be attained with 66.7% value bets and 33.3% bluffs including your worst hands. It could look like below:
What about different bet sizes?
Note that your bluffing percentage depends on the betting size. If you change your bet size, your range is also affected! Let’s consider that you value bet half the pot.
This time, your opponent will have to call a half pot bet to win three times as much, your bet, plus the pot. So, his pot odds are 3 to 1, and he would need to win 25% to break even. So, now your mix must contain 25% of bluffs to be balanced!
Note that, as long as you adopt a balanced range of bluffs and value bets, your EV is not affected by your bet size.
Game Theory Optimal Poker Software
How can you learn GTO poker?
There are many GTO solver software in the market, the most known are GTO+, PioSolver, Simple PostFlop, and GTO Range Builder that requires no download. Using these tools, you can set-up specific scenarios and run simulations. The solver goes through the exponential tree of how the hand can evolve in the different streets, and gives you an answer.
You can check out situations for which you do not know what a GTO balanced solution is and gradually start improving your skills. You can start by experimenting with these tools. Some offer a free version but allow you only to set up turn or river scenarios that require significantly less processing power to solve.
In a nutshell
There is a lot of fuzz around game theory optimal poker (GTO) over the last years, and in a way, this is justified. Understanding the principles of GTO can help your game greatly. It can show you what a balanced strategy is in different situations, help you improve your game by correcting leaks, and serve as a defense strategy against poker pros. However, the most you can gain out of GTO is understanding when opponents deviate from the baseline so that you can adapt accordingly. Since most players’ game is far from perfect, finding their shortcomings and playing exploitative poker will bring the most profit!
Game Theory Optimal vs. Exploitative Play in Poker
Poker is a game of probability, or so most people think. Given a hand, there is a certain probability of your opponent having a better hand. As a result, there is always an optimal decision to make that maximizes expected value. This style of play is called GTO (game theory optimal). Playing true GTO ensures that the best an opponent can do is break even. This sounds amazing, but it is not as good as it sounds. GTO completely ignores the fact that an opponent may have certain tendencies and given these tendencies, another style of play might yield higher expected value.
On the other hand, exploitative play exploits these tendencies. A player employing an exploitative style will identify and attack their opponent’s weaknesses. As a result, this style is higher variance. The very best poker players in the world play this style. In live poker tournaments, live reads are so important and the best players are able to identify weaknesses based on these reads. At the highest level, ability to play exploitative is synonymous with “skill”.
GTO vs. exploitative play can also be explained through the simple game of rock, pappers, scissors. A GTO approach would to play rock 33%, paper 33%, and scissors 33%. It is easy to see why this is hard to exploit. An exploitative strategy could employ a 43% rock, 23% paper, and 33% scissors. This exploits players that tend to use scissors more, but gets exploited by players who use paper more. Exploitative poker play is all about using this concept, but with the added information of opponent tendencies and weaknesses. As stated before, these tendencies and weaknesses are apparent in live poker games, where exploitative play tends to work better. GTO does have its merits–many great GTO players are extremely successful in online poker.
Source: https://www.upswingpoker.com/gto-vs-exploitative-play-game-theory-optimal-strategy/
Comments
Leave a Reply
Game Theory Optimal Play
Blogging Calendar
Optimal Game Theory Poker Download
| M | T | W | T | F | S | S |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | ||||
| 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 11 | 12 | 13 | 14 | 15 | 16 | 17 |
| 18 | 19 | 20 | 21 | 22 | 23 | 24 |
| 25 | 26 | 27 | 28 | 29 | 30 |